http://www.sciforums.com/threads/squaring-the-circle.141196/
http://www.sciforums.com/threads/help-needed.142484/ ( Discussion on construction of approximations to 1:√π including my discovery of 1 : ( √(3/10) + √(3/2) ) which is constructable )
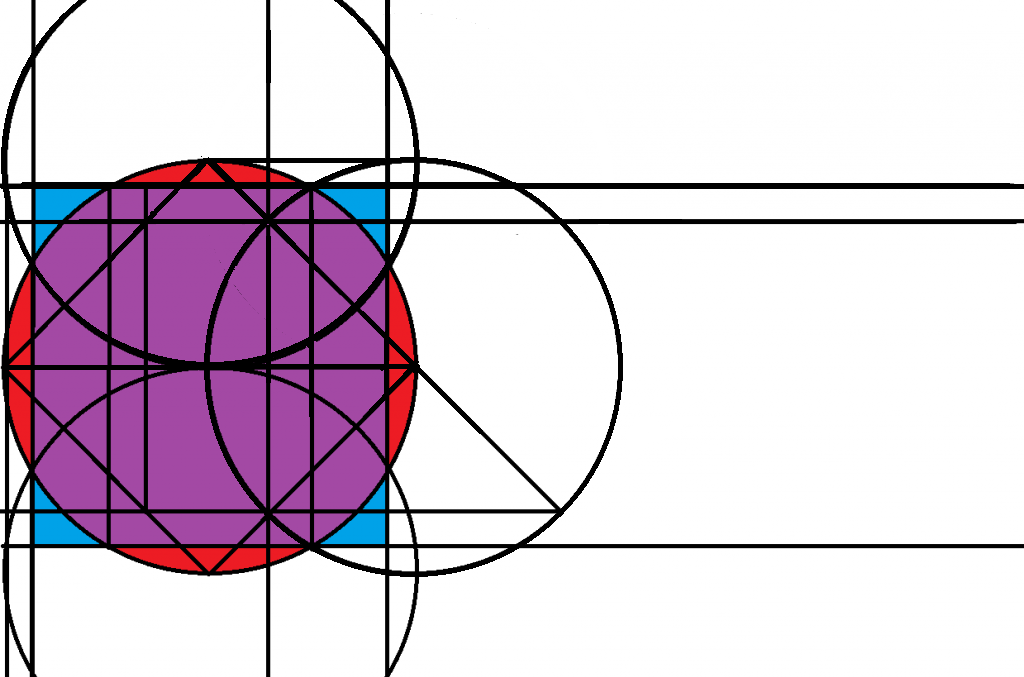
The diagram in post #106 is a humbug
It was originally posted in the context of a geometric construction of the ratio 1:√π but is not a geometric construction of 1:√π . If it is a geometric construction, it is for a square of side of length in ratio with the radius of the circle of 1:√3.
The diagram in post #106 is a humbug and any claim that it is a construction of a square with sides of length √3.14 or √π is a fraud. This is easiest to see in a cleaned up and labelled diagram:
View attachment 101
Here we see there is no connection between the trivially constructed length CD, with length in ratio AB:CD = 1:√3 and the red-bordered square WXYZ with sides in ratio AB:WX = 1:√π
This was Motor Daddy's burden to carry which he ignores.
View attachment 102
Zooming in we see that the point C is exactly defined by the intersection of two arcs and is quite a bit separated from the red line.