Where is most "gravity", inside or out?

[origin]

so here is my question: how would that play out, not in an ideal disk, but a sphere? , which is acting as a point mass close by, not only at a distance? could we have mis-interpreted gravity because overlooking (until now) this simple Ancient Greece arithmetic? Gravity's effects becoming more pronounced with distance?

The point is that a galaxy acts sort of like a solid. The gravity appears to fall on inside that galaxy in the vicinity of 1/r. One explanation (the one that most astronomers believe) is that there must be a tremendous amount of matter in the galaxy that only weakly interacts with matter. We already know of these types of particles (neutrinos) exist so it is not a stretch to imagine more massive particles that only very weakly interact with matter. It would be much more surprising if the issue turned out to be gravity itself, since we to not see any sort of issues on shorter distance scales. You can believe what you want but my money is on dark matter.

 

[nebel]

You can believe what you want but my money is on dark matter.

I am not a betting person, my question is, how does the diminishing increase in gravity affected area, according to the 2r x 3,14=circumference formula, explain the high velocity results we see? if any? if possibly? or not?--Occam's razor, it is a misreading of simple geometry?

 

[nebel]

The point is that a galaxy acts sort of like a solid.

that being the case, but not really being a solid, a galaxy spinning (discounting local anomalies) like an old fashioned vinyl disk, would that not allow for the comparatively lower inner velocities, and the higher outer? with zero gravity at the center?

 

[Janus58]

so, are you saying that dark matter is evenly distributed everywhere, even appearing in the expanding enclosed space of the universe? would that not mean that it would have zero effect on the existing gravity gradients ? , but only shift it to higher values ?
I am referring to the shell theorem, where an empty shell has zero gravity inside, only projecting it into the infinite distance, but with ever increasing effect, as opined in the thread on "gravity inside/outside". this because of the ever diminishing effects of linear expansion on the expanded perimeter. do not astrophysicists consider a distinct halo, wimpy or macho, as the source of the higher outer orbit velocities? PS : would ubiquitous dark matter not render the shell theorem invalid? dark matter not detected in recent experiments.

No, I said that the dark matter halo was a spherical mass in which the galaxy resided, I said nothing of it being evenly distributed everywhere. The halo extends past the visible galaxy but does fill all of space, it would look something like the blue region in this image

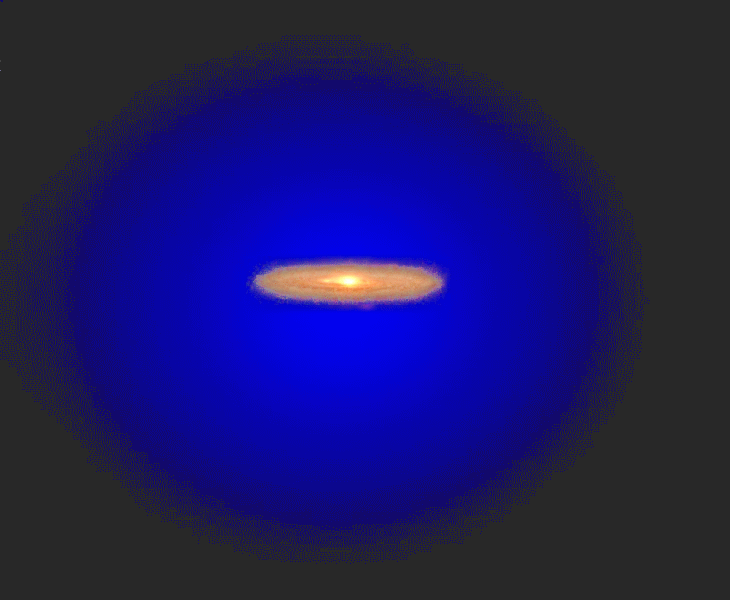
Also, it is not expected to be evenly distributed even in this region. There will a some increase in density towards the center.

The shell theorem states that a empty spherical shell has no gravity inside of it, but at no point is the dark matter halo an empty spherical shell. If I pick any given star, I can use it to divide the dark matter halo into two regions; That part further from the center and that part closer. While that part further away has no gravitational influence on the star as per the shell theorem, that part closer to the center does because the star is outside this volume of mass. ( it was this mass that I was referring to in my post above).

 

[nebel]

No, I said that the dark matter halo was a spherical mass in which the galaxy resided,

so, is the dark matter halo subject to the known gravity laws? no gravity at the center, and reaching out from the fuzzy perimeter, or it's maximum gravity radius to infinity? does dark matter rotate to keep from falling into the center?

 

[Janus58]

so, is the dark matter halo subject to the known gravity laws? no gravity at the center, and reaching out from the fuzzy perimeter, or it's maximum gravity radius to infinity? does dark matter rotate to keep from falling into the center?

For the dark matter halo, its net gravitational effect is zero at the center, then increases as you move outward(with the exact manner of the increase dependent on how the density of the dark matter decreases) until you reach the outer edge, then it decreases by the square cube law outside of the halo.

The components of dark matter do follow orbits around the barycenter of the galactic mass. However, these orbits are not as restricted as those for the visible matter. When the galaxy formed collisions between the visible matter elements cleared out the material that were orbiting in widely different directions or had extremely eccentric orbits. Since dark matter cannot collide with itself or visible matter, this mechanism didn't occur and its individual components can still orbit in all random directions and eccentricities. So you are going to get a more random distributions of motion than the more orderly orbits we see with the stars in the galaxy.

 

[nebel]

Since dark matter cannot collide with itself or visible matter, this mechanism didn't occur and its individual components can still orbit in all random directions and eccentricities. So you are going to get a more random distributions of motion than the more orderly orbits we see with the stars in the gala

so, would dark matter resemble the orbital situation that should prevail in open star clusters ? and how has this mechanism been observed, with dark matter not interacting with our equipment? and, if dark matter obeys the shell theorem, if in a halo, that outside component could not influence the situation, velocities on the inside of the galaxy, or?

 

[origin]

so, would dark matter resemble the orbital situation that should prevail in open star clusters ?

No that is completely different. An open star cluster is a temporary phenomena the new stars will 'go their own way' after some time due to interations with other stars not in the cluster.

and how has this mechanism been observed, with dark matter not interacting with our equipment?

With the effects of the dark matters gravity.

 

[nebel]

No that is completely different. An open star cluster is a temporary phenomena the new stars will 'go their own way' after some time due to interactions with other stars not in the cluster

sorry, I am learning here, I had meant to ask about "globular", not 'open' star clusters, those former, that have been around with longer lasting non-disk- star orbits. Have we been able to establish that dark matter follows those orbit patterns? and with the shell theorem governing dark matter too, how could the DM halo influence the gravity of the enclosed galaxy? thank you!

 

[origin]

sorry, I am learning here, I had meant to ask about "globular", not 'open' star clusters, those former, that have been around with longer lasting non-disk- star orbits. Have we been able to establish that dark matter follows those orbit patterns?

Not that I know of.

and with the shell theorem governing dark matter too, how could the DM halo influence the gravity of the enclosed galaxy? thank you!

The term DM halo can give the wrong impression. It is not a halo in the sense that there is no DM on the inside of the halo. The actual physical description is that there is a high density of DM in the center of the galaxy and there is a decreasing density as you move away from the center.
Like this:

 

[nebel]

here is a high density of DM in the center of the galaxy and there is a decreasing density as you move away from the center.

so, by your description, there is the same distribution of dark matter as there is of emitting baryonic matter? and: is dark matter subject to the shell theorem of gravity?

 

[origin]

so, by your description, there is the same distribution of dark matter as there is of emitting baryonic matter?

No. What in that picture I supplied would lead you to think that?

and: is dark matter subject to the shell theorem of gravity?

No. The shell theorem is about solid spheres or shell. Because of the large amount of dark matter the relationship for gravitational force approaches the shell theorem but it does not have the same relationship as a solid sphere.

 

[nebel]

Origin: "No. The shell theorem is about solid spheres or shell. Because of the large amount of dark matter the relationship for gravitational force approaches the shell theorem but it does not have the same relationship as a solid sphere.
The shell theorem does not require that the shells be solid, many shells are fuzzy, but it basically means that any symmetrical ball shaped outside mass has zero gravitational influence toward the center, so, that blob of dark matter does not matter for any attraction, velocities from any of it's inside loci, positions toward the center. all gravity is projected to the outside, from there to eternity. all matter , dark or shiny. or?

 

[nebel]

origin, in any sphere, you could produce an imaginary interior onion -like layer. Each of these smaller spheres will have zero gravity toward the inside, and project all their gravity, (gravitons, spacetimetension,whatever it turns out to be) to the eternal exterior. That is how gravity falls to zero at the center, only ever smaller spheres available to fill their exteriors with the pull of the smaller masses. that is why propose the wording:
There is no gravity toward the inside, only on the outside.---or?

 

[origin]

origin, in any sphere, you could produce an imaginary interior onion -like layer. Each of these smaller spheres will have zero gravity toward the inside, and project all their gravity, (gravitons, spacetimetension,whatever it turns out to be) to the eternal exterior. That is how gravity falls to zero at the center, only ever smaller spheres available to fill their exteriors with the pull of the smaller masses. that is why propose the wording:
There is no gravity toward the inside, only on the outside.---or?

The galaxy is not spherical shell or a sphere with approximately constant density at a given radius so it does not follow shell theorem exactly. As a matter of fact when you are very close to the center of the galaxy you will actually feel the greatest gravitational force orce.

 

[Janus58]

Origin: "No. The shell theorem is about solid spheres or shell. Because of the large amount of dark matter the relationship for gravitational force approaches the shell theorem but it does not have the same relationship as a solid sphere.
The shell theorem does not require that the shells be solid, many shells are fuzzy, but it basically means that any symmetrical ball shaped outside mass has zero gravitational influence toward the center, so, that blob of dark matter does not matter for any attraction, velocities from any of it's inside loci, positions toward the center. all gravity is projected to the outside, from there to eternity. all matter , dark or shiny. or?

The shell theorem states that a uniform hollow shell has no gravitational effect on its interior. It does not state that mass inside of the shell has no gravitational effect.

Fig. 1 below shows a sphere of some mass. If you place a test mass on its surface it will attracted towards the center of the sphere by a certain magnitude of gravity.
If you fit a hollow shell around this sphere as in Fig 2, The extra mass, as per the shell theorem has no gravitational effect on it interior, but it doesn't cancel out the gravitational effect that it already there. That same test mass, sitting where the inner sphere and outer shell meet, still feels the same force of gravity pulling it towards the center of the sphere as it did before it was enclosed with the shell.
Assuming that the density of the shell is the same as the sphere, if we put the test mass on the outer surface of the shell, it is twice as far from the center, but the combined mass of the shell and sphere is 8 times that of the sphere alone. The net result is that the test mass will feel twice the gravitational force that is did sitting on the surface of the inner sphere.

If you keep adding shells, (or divide a sphere up into shells) as in Fig. 3, you get the same effect. Put a test mass anywhere in it, and while it feels no gravitational effect form the mass of the shells exterior to it, it does feel a gravitational effect from the mass of all the shell interior to it.

Fig. 4 show a representation of a galaxy inside its dark matter halo. If you were just to consider the galaxy and worked out the gravitational effect as you move out from the center, you would expect things to work out pretty much the same as above with gravity increasing as you moved outward from the center*. However, once you get out into the disk, the interior mass at any point now longer increases by the cube of the distance, and the increasing distance begins to dominate causing a fall-off of gravity as you continue to move outward. In terms of orbital velocities, you would see an increase at you moved outward while in the bulge, and then a decrease as you moved outward once in the disk region.

When you add the dark matter halo (as shown in Fig. 4) you also have to take into account the additional mass of the shells of dark matter interior to your position. Even once you move outward into the disk of the galaxy, the volume including this mass increases by the cube of the distance. Now the density of the dark matter halo is quite low compared to that of the galaxy itself, so while you are in the bulge, this additional mass doesn't have much of an effect. But once you get into the disk, the mass of the dark matter in the interior shells adds up much faster than the added mass of stars in the disk as you move out, and it exerts a greater and greater relative influence. This influence is to flatten out the decrease in orbital speed as you move out from the center to the point where it is close to non-existent, and the velocities hardly decrease at all (or in some cases actually increase).

Now the dark matter halo extents well past the galaxy itself, and those shells further out than the edge of the disk have no effect on the galaxy. So how do we know its there? Galaxies are rarely solitary for one thing. Our own galaxy has satellite dwarf galaxies orbiting it. So we can look at these and their velocities to get an idea of how the dark matter is distributed outside of the galaxy. In addition, gravity bends the path of light, so by looking at how light is lens as it passes through the regions exterior to the visible part of the galaxy we can map the dark matter halo.

* Here we are assuming a fairly constant density or at least a density that increases smoothly as you approach the center of the galaxy. This isn't exactly true, because we have very high density object in the form of a super-massive black hole at the center. Once you start getting close to it, it will begin to dominate and gravity force goes up. We actually see something of the same effect for the Earth. The crust is much less dense than the interior, and as a result, as you move from the surface towards the interior, for the first few miles or so the force of gravity will increase before it starts to decrease again.

 

[origin]

The shell theorem states that a uniform hollow shell has no gravitational effect on its interior. It does not state that mass inside of the shell has no gravitational effect.

Fig. 1 below shows a sphere of some mass. If you place a test mass on its surface it will attracted towards the center of the sphere by a certain magnitude of gravity.
If you fit a hollow shell around this sphere as in Fig 2, The extra mass, as per the shell theorem has no gravitational effect on it interior, but it doesn't cancel out the gravitational effect that it already there. That same test mass, sitting where the inner sphere and outer shell meet, still feels the same force of gravity pulling it towards the center of the sphere as it did before it was enclosed with the shell.
Assuming that the density of the shell is the same as the sphere, if we put the test mass on the outer surface of the shell, it is twice as far from the center, but the combined mass of the shell and sphere is 8 times that of the sphere alone. The net result is that the test mass will feel twice the gravitational force that is did sitting on the surface of the inner sphere.

If you keep adding shells, (or divide a sphere up into shells) as in Fig. 3, you get the same effect. Put a test mass anywhere in it, and while it feels no gravitational effect form the mass of the shells exterior to it, it does feel a gravitational effect from the mass of all the shell interior to it.

Fig. 4 show a representation of a galaxy inside its dark matter halo. If you were just to consider the galaxy and worked out the gravitational effect as you move out from the center, you would expect things to work out pretty much the same as above with gravity increasing as you moved outward from the center*. However, once you get out into the disk, the interior mass at any point now longer increases by the cube of the distance, and the increasing distance begins to dominate causing a fall-off of gravity as you continue to move outward. In terms of orbital velocities, you would see an increase at you moved outward while in the bulge, and then a decrease as you moved outward once in the disk region.

When you add the dark matter halo (as shown in Fig. 4) you also have to take into account the additional mass of the shells of dark matter interior to your position. Even once you move outward into the disk of the galaxy, the volume including this mass increases by the cube of the distance. Now the density of the dark matter halo is quite low compared to that of the galaxy itself, so while you are in the bulge, this additional mass doesn't have much of an effect. But once you get into the disk, the mass of the dark matter in the interior shells adds up much faster than the added mass of stars in the disk as you move out, and it exerts a greater and greater relative influence. This influence is to flatten out the decrease in orbital speed as you move out from the center to the point where it is close to non-existent, and the velocities hardly decrease at all (or in some cases actually increase).

Now the dark matter halo extents well past the galaxy itself, and those shells further out than the edge of the disk have no effect on the galaxy. So how do we know its there? Galaxies are rarely solitary for one thing. Our own galaxy has satellite dwarf galaxies orbiting it. So we can look at these and their velocities to get an idea of how the dark matter is distributed outside of the galaxy. In addition, gravity bends the path of light, so by looking at how light is lens as it passes through the regions exterior to the visible part of the galaxy we can map the dark matter halo.

* Here we are assuming a fairly constant density or at least a density that increases smoothly as you approach the center of the galaxy. This isn't exactly true, because we have very high density object in the form of a super-massive black hole at the center. Once you start getting close to it, it will begin to dominate and gravity force goes up. We actually see something of the same effect for the Earth. The crust is much less dense than the interior, and as a result, as you move from the surface towards the interior, for the first few miles or so the force of gravity will increase before it starts to decrease again.

As usual you have presented an excellent post, thank you.

 

[nebel]

those shells further out than the edge of the disk have no effect on the galaxy.

excellent post, just can not see the figures 1-4. that is the point: any outside mass has no effect on the interior. the gravitational effect is all outside. it is not a shell game: there is no gravity in any of these peel - away shells that originates in the outside. or?

 

[The God]

.... As a matter of fact when you are very close to the center of the galaxy you will actually feel the greatest gravitational force orce....

Not correct in general. For uniform density spherical mass distribution which is simplest form, the force increases linearly as you move away from the center......for all other configuration also it is actually m/r^2 that determines the relationship.

 

[origin]

Not correct in general.

Of course not, but it is correct in this specific case which is what the question was about.